Anthony Leverrier: SU(p,q) coherent states and Gaussian de Finetti theorems

Опубликовано 1 февраля 2017, 1:40

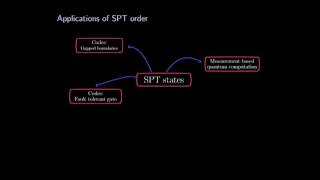
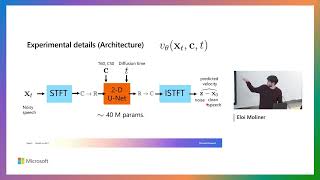
We prove generalizations of de Finetti theorems where the local space is not finite-dimensional but rather an infinite-dimensional Fock space. In particular, instead of considering the action of the permutation group on $n$ copies of that space, we consider the action of the unitary group $U(n)$. We define a natural generalization of the symmetric subspace as the subspace that is invariant under unitaries in $U(n)$. We show that this subspace is spanned by a family of generalized coherent states related to the Lie group $SU(p,q)$. More precisely, these (Gaussian) states resolve the identity on the subspace, which establishes a de Finetti theorem. Our theorem finds an important application in the context of quantum cryptography with continuous variables, since it allows us to prove a tight reduction from general attacks to Gaussian collective attacks, which are far easier to analyze. In particular, this establishes the security of some continuous-variable quantum key distribution with (standard Glauber) coherent states, in the finite-size regime.